PICWave
PICWave is a Photonic Circuit (PIC) simulator including a sophisticated physics model for laser diodes and SOAs. The simulator is ideal for developing optoelectronic devices such as tunable lasers and modulators and then studying their interaction in a larger circuit.
Request a demo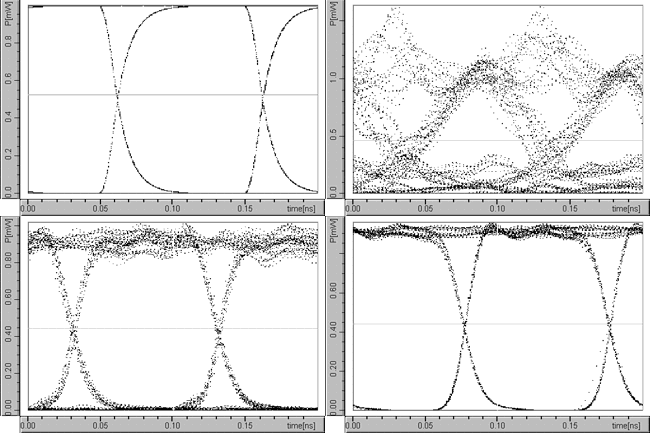
Applications
- Photonic integrated circuit (PIC) design
- Laser diode design and SOA
- Hybrid silicon lasers
- Lasers DFB
- Hybrid silicon lasers
- Ring resonators
- Modulators
- Photodetectors
- JePPIX Platform Design Kits
- PDAFlow export
Request a demo
Examples
- SOA modeling (link with Harold)
-
Laser locked in mode
-
Quarter-wave shifted DFB laser + modulator
-
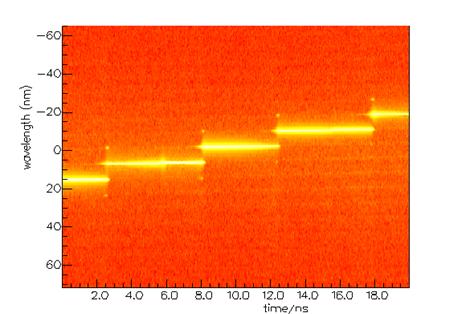
SG-DBR tunable laser
-
SOI hybrid cone laser
-
Mode hopping in a Fabry-Perot laser
-
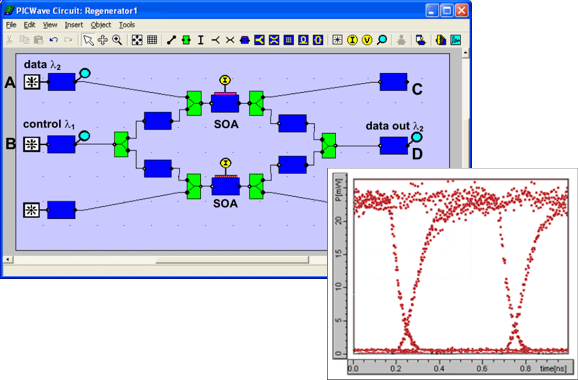
Active optical 2R regenerator
-
Cx4 optical switch
-
Balanced photodetector
-
Tunable wave modulator
-
Nonlinear processes in periodically-poled lithium niobate
- Large ring resonators (related to FIMMPROP)
-
Dispersion-compensating fiber Bragg grating
Request a demo
Optional Modules for PICWave
Active Module
This module enables the simulation of charge carrier effects in active materials, supporting the modeling of various active devices such as SOAs, laser diodes, and even multi-section tunable lasers. It also includes an electro-absorption modulator model.
Complex Engine
The Complex Engine adds the ability to solve metallic structures rigorously and account for the influence of gain or loss on mode shapes. This is particularly useful for large, weakly guided modes, such as those in high-power, low-confinement-factor lasers. Additionally, it introduces PMLs for leaky mode computations.
Kallistos
An advanced automatic optimization tool that enhances the design of photonic devices with minimal user intervention.
Connected Products
Harold
The hetero-structure model Harold generates gain curves for PICWave. These curves can be fitted using PICWave’s Wide-Band Gain Model, enabling accurate gain spectrum modeling across a broad wavelength range. Gain spectra from other sources can also be incorporated.
FIMMPROP
FIMMPROP facilitates rigorous modeling of passive photonic components, which can then be included in PICWave circuit simulations.
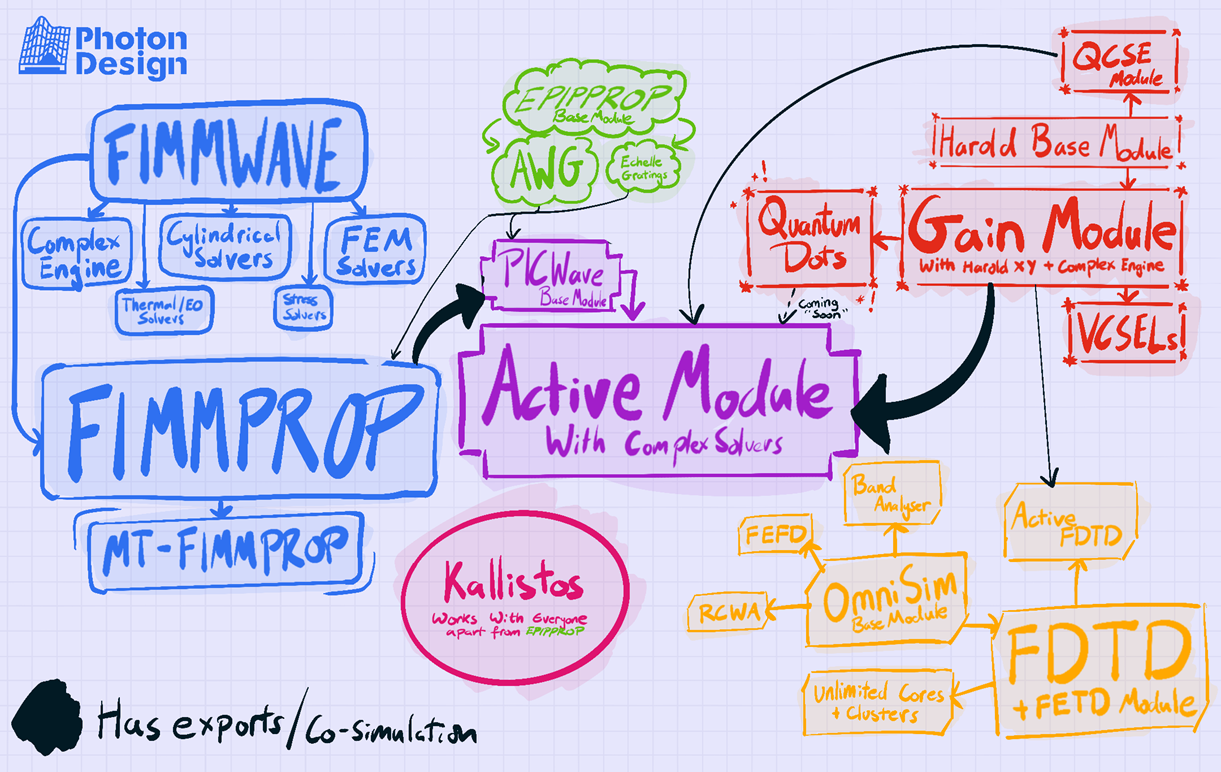
Photon Design Module map
This module map provides a visual overview of the Photon Design software suite, showing how the different tools connect to support your integrated photonics simulations. It starts with the core FIMMPROP module and includes key combinations such as Harold and PICWave. The size of each module indicates its relative role in the design process, while the black lines represent the data flow between components. Together, these tools form a cohesive environment for designing and optimizing photonic devices.
Master PICWAVE with Our Video Library
Discover the power of PICWAVE with our video guides. Whether you’re new or experienced, our videos will help you unlock the full potential of PICWAVE features and capabilities.
Latest Updates
Stay up to date with the latest features and improvements in PICWave. Below are the most recent updates that enhance your photonics design and simulation experience.
